Does the object represented in Figure Q1.5 have a positive or negative value of 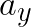 ? Explain.
? Explain.

Figure Q1.5
Continue reading

Figure Q1.5

Figure Q1.4

Figure Q1.3
The diagram does not indicate any position in time that should have been represented by numbers on the dots. Without numbers on the dots we cannot tell if the particle in the figure is moving left or right, so we can’t tell if it is speeding up or slowing down. If the particle is moving to the right it is speeding up. If it is moving to the left it is slowing down.
Part a
Zeroes before the decimal point merely locate the decimal point and are not significant. Thus, 310 has 2 significant figures.
Part b
Trailing zeros after the decimal point are significant because they indicate increased precision. Therefore, 0.00310 has 3 significant figures.
Part c
Zeroes between nonzero digits are significant. Therefore, 1.031 has 4 significant figures.
Part d
Just like in part b, trailing zeros after the decimal point are significant because they indicate increased precision.Therefore, 3.10×105 has 3 significant figures.
Part a
All nonzero digits are significant, therefore, 53.2 has 3 significant figures.
Part b
The number 0.53 can be written in scientific notation as 5.3×10-1. Therefore, 0.53 has 2 significant figures.
Part c
Trailing zeros are significant because they indicate increased precision. Therefore, 5.320 has 4 significant figures.
Part d
The leading zeros are not significant but just locate the decimal point. Therefore, 0.0532 has only 3 significant figures.
Conceptual Questions
Exercises and Problems
Question 29
Question 30
Question 31
Question 32
Question 33
Question 34
Question 35
Question 36
Question 37
Question 38
Question 39
Question 40
Question 41
Question 42
Question 43
Question 44
Question 45
Question 46
Question 47
Question 48
Question 49
Question 50
Question 51
Question 52
Question 53
Question 54
Question 55
Question 56
Question 57
Learning Goal:

To subtract B⃗ from A⃗ (Figure 1), perform these steps:











SOLUTION:
Draw the free-body diagram of the car

Consider the vertical direction
Consider the motion in the horizontal direction
Solve for the acceleration of the car.
Solve for the coefficient of kinetic friction
SOLUTION:
The free-body diagram of the box

Solve for the normal force
Solve for the friction force
Solve for the acceleration in the horizontal direction
Therefore, the acceleration of the box is 6.51 m/s2 along the horizontal surface.
You must be logged in to post a comment.